Question

The equation x^3-5x=59 has a solution between 4 and 5. Use a trial and improvement method to find this solution. Give your answer to one decimal place. You must show all your working. On the same line as your final answer, you must write to "1 decimal place".

Answer 1

The answer is "4.3".

Explanation:

Given:

`\to x^3 - 5x - 59 = 0`

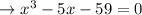
When
`x = 4.1:`

`\to f(4.1) = (4.1)^3 - 5(4.1) - 59 = -10.579`

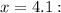
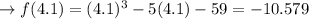
When
`x= 4.2:`

`f(4.2) = 4.2^3 - 5(4.2) - 59 = -5.912`

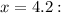
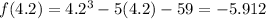
When
`x = 4.3:`

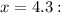
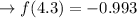`\to f(4.3) = -0.993` (closer to 0)

`\to f(4.4) = 4.184`

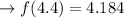
• It changes the sign so, the root lies in 4.3 and 4.4.

When
`x = 4.35:`

`\to f(4.35) = 1.563`

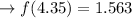
When the root is closer to 4.3 than 4.4 So, 1.563 is closer to zero than 4.184.

When
`x = 4.32:`

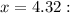
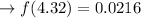`\to f(4.32) = 0.0216` close to zero.

So the answer is 4.3 to 1 decimal place.