Question

Find the surface area of a square pyramid with side length 6 cm and slant height 7 cm.

Answer 1

120

Explanation:

Over all plan:

This is a square pyramid so you know that all four triangular sides are congruent. This means that to solve this problem, which is asking you to find the surface area, you need to find the area of one of these triangles, and multiply that by 4. Next you need to find the area of the square and add it to the areas of the triangles.

Detailed Steps:

• You know the formula to finding the area of the triangle is
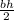  `(bh)/(2)` where b is the base length and h is the height. We are given b = 6 and h = 7 in your question.

1. Plug in the values into the formula. You get:
   `(6\cdot7)/(2)`
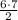
2. Simplify. You get:
   `(42)/(2) = 21`

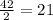
• Next Multiply this by 4 to find out the total area of all the triangular faces.

1. 21 x 4 = 84

• Now find the area of the square.

1. The formula for the area of a square is
   `s^2` where s is the given value of a side.

Plug in your value,
`6^2=36`

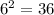
36+84=120.

120 is your final answer.

hope this helped

peace ✌