Question

In a recent sample of 84 used car sales costs, the sample mean was $6,425 with a standard deviation of $3,156. Assume the underlying distribution is approximately normal.a. Which distribution should you use for this problem

Answer 1

By the Central Limit Theorem, an approximately normal distribution, with mean $6425 and standard deviation $344.35.

Explanation:

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

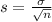For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

Sample mean was $6,425 with a standard deviation of $3,156

This means that
`\mu = 6425, \sigma = 3156`

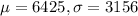
Sample of 84:

This means that
`n = 84, s = (3156)/(√(84)) = 344.35`

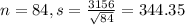
a. Which distribution should you use for this problem?

By the Central Limit Theorem, an approximately normal distribution, with mean $6425 and standard deviation $344.35.