Question

What value of x and y makes the 2 triangles congruent by ASA? Find the measures of angle QPR and QRP

Answer 1

Given:

Two triangles congruent by ASA.

To find:

The the measures of x, y, angle QPR and angle QRP.

Solution:

The triangles PQR and PSR are congruent by ASA. So,

`\angle QPR\cong \angle SPR`

`PR\cong PR`

`\angle QRP\cong \angle SRP`

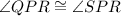
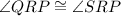
Now,

`m\angle QPR=m\angle SPR`

`2x+1=x+18`

`2x-x=18-1`

`x=17`

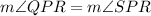
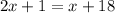
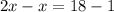
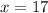
And,

`\angle QRP= \angle SRP`

`8y-4=4y+28`

`8y-4y=4+28`

`4y=32`

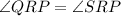
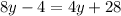
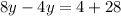
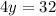
Divide both sides by 4.

`y=8`

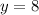
The measure of angle QPR is:

`m\angle QPR=(2x+1)^\circ`

`m\angle QPR=(2(17)+1)^\circ`

`m\angle QPR=(35+1)^\circ`

`m\angle QPR=36^\circ`

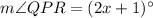
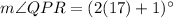
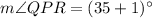
And,

`m\angle QRP=(8y-4)^\circ`

`m\angle QRP=(8(8)-4)^\circ`

`m\angle QRP=(64-4)^\circ`

`m\angle QRP=60^\circ`

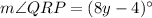
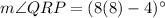
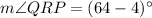
Therefore, the required values are
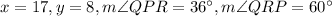`x=17, y=8, m\angle QPR=36^\circ ,m\angle QRP=60^\circ`.