Answer:

Explanation:
Geometric sequence:
In a geometric sequence, the quotient between consecutive terms is the same, and this quotient is given by q.
The explicit rule of a geometric sequence is given by:
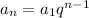
In which
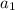 is the first term.
is the first term.
1/4, 1/2, 1, 2, 4
This means that
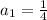 , and:
, and:
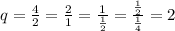
So the explicit rule is:
