Question

What is the slope of the line that is parallel to the line joining the points (7, -5) and (-5, 7)?

Answer 1

The slope is -1.

Explanation:

We want to find the slope of the line that is parallel to the line joining the points (7, -5) and (-5, 7).

First, we can use the slope formula to find the line passing through (7, -5) and (-5, 7). The slope formula is:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

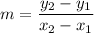
Substitute and evaluate:

`\displaystyle m=((7)-(-5))/((-5)-(7))=(12)/(-12)=-1`

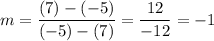
So, its slope is -1.

Recall that parallel lines have the same slope.

Therefore, the slope of the line that is parallel to the line joining the points (7, -5) and (-5, 7) will also be -1.