Question

Patricia and Whitney began arguing about who did better on their tests, but they couldn't decide who did better given that they took different tests. Patricia took a test in English and earned a 76.5, and Whitney took a test in Math and earned a 64.5. Use the fact that all the students' test grades in the English class had a mean of 73.1 and a standard deviation of 10.5, and all the students' test grades in Math had a mean of 60.9 and a standard deviation of 10.7 to answer the following questions.

a. Calculate the Z-score for Kimberly's test grade.
b. Calculate the z-score for Karina's test grade.
c. Which person did relatively better?

Answer 1

a) The Z-score for Patricia's test grade is 0.32.

b) The z-score for Whitney's test grade is of 0.34.

c) Due to the higher z-score, Whitney's did relatively better.

Explanation:

Z-score:

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

a. Calculate the Z-score for Patricia's test grade.

Patricia took a test in English and earned a 76.5. Students' test grades in the English class had a mean of 73.1 and a standard deviation of 10.5.

This means that
`X = 76.5, \mu = 73.1, \sigma = 10.5`

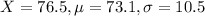
The z-score is:

`Z = (X - \mu)/(\sigma)`

`Z = (76.5 - 73.1)/(10.5)`

`Z = 0.32`

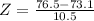
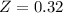
The Z-score for Patricia's test grade is 0.32.

b. Calculate the z-score for Whitney's test grade.

Whitney took a test in Math and earned a 64.5. Students' test grades in Math had a mean of 60.9 and a standard deviation of 10.7.

This means that
`X = 64.5, \mu = 60.9, \sigma = 10.7`

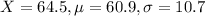
The z-score is:

`Z = (X - \mu)/(\sigma)`

`Z = (64.5 - 60.9)/(10.7)`

`Z = 0.34`

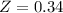
The z-score for Whitney's test grade is of 0.34.

c. Which person did relatively better?

Due to the higher z-score, Whitney's did relatively better.