Question

Hello help me with this question thanks in advance​

Answer 1

#3 Answer:

The other sides are 18.75 and 22.5

#3 Step-by-step explanation:

Because of the similar triangle

So,
`(8)/(10)=(15)/(x)=(18)/(y)`
`\left\{The\ corresponding\ sides\ are\ proportional\right\}`

`x=18.75,\ y=22.5`

#4 Answer:

4:5

#4 Step-by-step explanation:

`(4)/(5)=(12)/(15)=(20)/(25)=(6)/(5)`

`So\ the\ scale\ factor\ is\ 4:5`

#5 Answer:

24 cm

#5 Step-by-step explanation:

`12/0.5`

Calculate

`(12)/(0.5)`

Multiply both the numerator and denominator with the same integer

`(120)/(5)`

Cross out the common factor

24

I hope this helps you

:)

Answer 2

`\bold{\huge{\underline{ Solution \:1}}}`

Here, We have given

• The sides of a triangle are 8 , 15 and 18
• The one of the side of the similar traingle is 10.

Let assume the given triangle as ΔABC and ΔXYZ

According to the similarity theorem

• If two triangle's are similar then the ratio of their corresponding sides are also equal .

Therefore, By using above theorem :-

`\sf{( A)/(X)}{\sf{=}}{\sf{( B)/(Y)}}{\sf{=}}{\sf{( C)/(Z)}}`

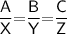
Subsitute the required values

`\sf{( 8)/(10)}{\sf{=}}{\sf{( 15 )/(Y)}}{\sf{=}}{\sf{(18)/(Z)}}`

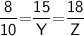
For other two sides of another similar triangle

`\sf{( 8 )/(10)}{\sf{=}}{\sf{( 15)/(Y)}}`

`\sf{Y =15}{\sf{*{( 10)/(8)}}}`

`\sf{Y =15}{\sf{*{( 5)/(4)}}}`

`\sf{Y = }{\sf{( 75)/(4)}}`

`\bold{Y = 18.75 }`

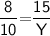
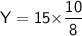
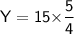
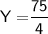
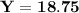
And,

`\sf{( 8 )/(10)}{\sf{=}}{\sf{( 18)/(Z)}}`

`\sf{Z =18 }{\sf{*{( 10)/(8)}}}`

`\sf{Z =18}{\sf{*{( 5)/(4)}}}`

`\sf{Z = }{\sf{( 90)/(4)}}`

`\bold{Z = 22.5 }`

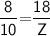
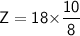
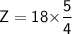
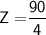
Hence, The two sides of the another similar triangle is 18.75 and 22.5

`\bold{\huge{\underline{ Solution \:2}}}`

Here, We have

• Two similar triangles
• Whose sides are 4 , 12 ,20 and 5 , 15 , 25

Let assume the two triangle be ΔABC and ΔPQR

According to the similarity theorem :-

• If two triangle's are similar then the ratio of their corresponding sides are also equal .

That is,

`\sf{( A)/(P)}{\sf{=}}{\sf{( B)/(Q)}}{\sf{=}}{\sf{( C)/(R)}}`

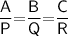
Subsitute the required values,

`\sf{(4 )/(5)}{\sf{=}}{\sf{( 12)/(15)}}{\sf{=}}{\sf{( 20 )/(25)}}`

`\sf{(4 )/(5)}{\sf{=}}{\sf{\cancel{( 12)/(15)}}}{\sf{=}}{\sf{\cancel{( 20 )/(25)}}}`

`\bold{(4 )/(5)}{\sf{=}}{\bold{( 4)/(5)}}{\sf{=}}{\bold{( 4 )/(5)}}`

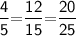
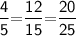
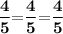
Hence, The scale factor of the given similar triangles is 4/5

`\bold{\huge{\underline{ Solution \: 3}}}`

Here, we have given that

• A map in Davao City has a scale factor of 1 cm to 0.5 km
• That is,
• 1 : 0.5

But,

• We have to find the distance corresponds to an actual distance of 12 km.

Therefore,

According to the scale factor

The actual distance will be

`\sf{=}{\sf{( 12 )/(0.5)}}`

`\bold{ = 24 \: km }`

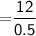
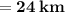
Hence, The map distance corresponds to actual distance of 12 km is 24 km.