Question

Find the gradient of the line passing through the points (4a,-a) and (6a,5a)

Answer 1

The gradient of the line passing through the points (4·a, -a) and (6·a, 5·a) is 3

Explanation:

The gradient of a (straight) line given the 'x' and 'y' coordinates of two points on the line, (x₁, y₁), and (x₂, y₂) can be found using the following formula;

`The \ gradient \ of \ a \ line, \, m =(y_(2)-y_(1))/(x_(2)-x_(1))`

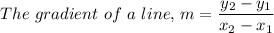
The coordinates of two points on the given line are;

(4·a, -a), and (6·a, 5·a)

Therefore, we get;

`The \ gradient \ of \ the \ line =(5\cdot a-(-a))/(6 \cdot a-4 \cdot a) = (6\cdot a)/(2 \cdot a) = 3`

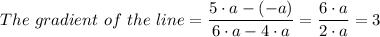
The gradient of the line = 3.