Answer:
The ratio of the new volume to the old volume is 8 to 1.
Step-by-step explanation:
Let's first list what we know:
- A cube has sides with lengths of 4 m
- The volume of the cube is 64
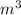
- The new cube has sides double the length of the old cube
Since the new cube has sides double the length of the sides of the old cube, and 4 doubled is 8, the length of the sides of the new cube is 8.
The equation for the volume of a cube is
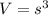 , where "V" is the volume and "s" is the lengths of the sides.
, where "V" is the volume and "s" is the lengths of the sides.
Now, let's solve for the volume of the new cube:
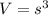
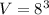
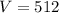
The volume of the new cube is 512
 .
.
The ratio of the new volume to the old volume is 512 : 64.
Let's simplify the ratio:
512 : 64
8 : 1
The ratio of the new volume to the old volume is 8 to 1.
P.S. This question should be in the mathematics subject, not the physics subject. (I pretty much only do math problems, so yes, it does matter. I don't know about the others though.)