Given:
The length of your family's garden is 3 feet greater than the width.
The area of the garden is 460 square feet.
To find:
The dimensions of the garden.
Solution:
Let x feet be the width of the garden. Then,
Length =
 feet
feet
The area of a rectangle is:
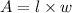
Where, l is the length and w is the width of the rectangle.
The area of the rectangular garden is:
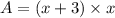
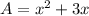
It is given that the area of the garden is 460 square feet.
Putting
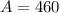 , we get
, we get
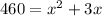
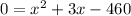
Splitting the middle term, we get

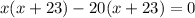

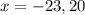
The width of a garden cannot be negative. So,
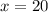 .
.
Now,
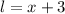
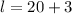
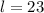
Therefore, the length of the garden is 23 feet and the width of the garden is 20 feet.