Answer:
(a)
 and
and
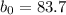
(b)
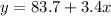
(c)
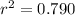
(d)
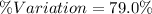
(e) The expected earnings is: $110,900
Step-by-step explanation:
The data for the 10 sales person is as follows:
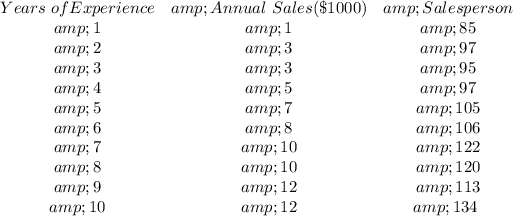
Required
Perform a regression analysis using Microsoft Excel
Note
- I added two attachments to this solution
- In the first attachment, I highlighted the step to follow to perform regression analysis.
- At step 4, ensure that you fill in the input y range and the input x range.
- The input y range (in this question) is the column for sales person while the input x range is the annual sales
- Click ok, then Microsoft Excel will perform the analysis for you.
The question will be answered based on the result of the analysis performed by the application (See attachment 2)
See attachment 2 for the result of the analysis (I've highlighted the solution to each question on the attached file)
(a) b1 and b0
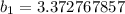
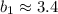
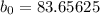
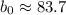
(b) The estimated regression equation
The equation is of the form:
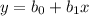
Where:

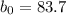
So:
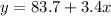
(c) The coefficient of determination (r^2)
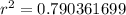
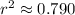
(d) Percentage of variation
To do this, we simply convert r^2 to percentage
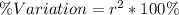
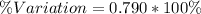
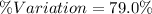
(e) Expected annual sales of a sales person with 8 years of experience.
Using the regression equation
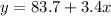
Where
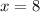 --- the years of experience.
--- the years of experience.
So;
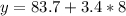
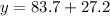
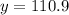
The expected earnings is: $110,900