Question

A projectile is fired vertically upward, and its height s(t) in feet after t seconds is given by the function s(t)=-16t^2+600t+800. After how many seconds, to the nearest tenth, will the projectile hit the ground?

Answer 1

The projectile will hit the ground after 38.8 seconds.

Explanation:

You know that the height s (t) in feet of the projectile after t seconds is given by the function

s(t) = - 16t² + 600t + 800.

To know the time at which the projectile will hit the ground, you must replace s(t) by height 0:

0=- 16t² + 600t + 800

This is a quadratic function of the form ax2 + bx + c, where a= -16, b=600 and c=800.

To solve a quadratic function, you must apply the expression:

`x1,x2=\frac{-b+-\sqrt{b^(2)-4*a*c } }{2*a}`

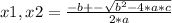
In this case:

`x1,x2=\frac{-600+-\sqrt{600^(2)-4*(-16)*800 } }{2*(-16)}`

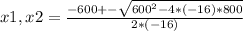
Solving:

`x1,x2=(-600+-√(360000+51200 ) )/(-32)`

`x1,x2=(-600+-√(411200 ) )/(-32)`

`x1,x2=(-600+-641.25 )/(-32)`

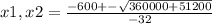
So:

`x1=(-600+641.25 )/(-32)`

`x1=(41.25 )/(-32)`

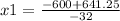
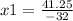
x1= -1.289 seconds

and

`x2=(-600-641.25 )/(-32)`

`x2=(-1241.25 )/(-32)`

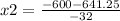
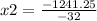
x2= 38.789 ≅ 38.8 seconds

Time cannot be negative. So, the projectile will hit the ground after 38.8 seconds.