Answer:
The equation is
 -
-
Explanation:
According to the statement, we have a second order polynomial of the form
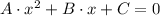 , whose solutions can be found by Quadratic Formula:
, whose solutions can be found by Quadratic Formula:
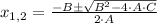 (1)
(1)
Where
 ,
,
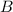 and
and
 are the coefficients of the polynomial.
are the coefficients of the polynomial.
If roots are conjugated complex numbers, then:
 (2)
(2)
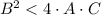
If we know that
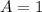 ,
,
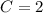 and
and
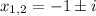 , then we find that:
, then we find that:
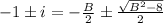
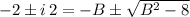
By comparing each side, we have the following system of equations:
 (3)
(3)
 (4)
(4)
Whose solution is
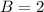 .
.
In a nutshell, the equation is
 -
-