Question

A wave pulse travels along a stretched string at a speed of 100 cm/s. What will be the speed in cm/s if the string's tension is quadrupled, the length halved and its mass is doubled

Answer 1

The new velocity of the string is 100 centimeters per second (1 meter per second).

Step-by-step explanation:

The speed of a wave through a string (
`v`), in meters per second, is defined by the following formula:

`v = \sqrt{(T\cdot L)/(m) }` (1)

Where:

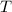`T` - Tension, in newtons.

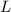`L` - Length of the string, in meters.

`m` - Mass of the string, in kilograms.

The expression for initial and final speeds of the wave are:

Initial speed

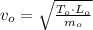`v_(o) = \sqrt{(T_(o)\cdot L_(o))/(m_(o)) }` (2)

Final speed

`v = \sqrt{((4\cdot T_(o))\cdot (0.5\cdot L_(o)))/(2\cdot m_(o)) }`

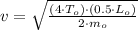
`v = \sqrt{(T_(o)\cdot L_(o))/(m_(o)) }` (3)

By (2), we conclude that:

`v =v_(o)`

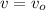
If we know that
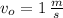`v_(o) = 1\,(m)/(s)`, then the new speed of the wave in the string is
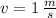`v = 1\,(m)/(s)`.