Hello!
This is a problem about relating circle theorems to line lengths.
We can first see that both line segment MK and CM are secants within the circle that come from a common point K.
This means that the Intersecting Secant Theorem applies here.
The Intersecting Secant Theorem states that if two secants are formed from a common point outside the circle, the length of each secant multiplied by the length of its corresponding external secant are equivalent.
We can set up the following equation.
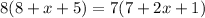
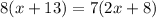
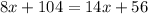
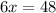
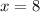
Using this value, we can find the length of line segment MK.

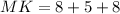
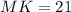
Hope this helps!