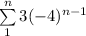Question

Please help very urgent

Answer 1

(a)

`T_n = 3(-4)^(n-1)`

(b)

`\sum\limits^n_1 { 3(-4)^(n-1)`

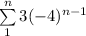
Explanation:

Given

The above sequence

Solving (a): The explicit formula

The given sequence is a geometric sequence because the common ratio (r) is:

`r = (-12)/(3) = (48)/(-12) = (-192)/(48) = (768)/(-192)`

`r = -4`

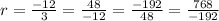
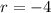
The explicit formula is calculated using the n term of an GP.

`T_n = ar^(n-1)`

`T_n = 3 * (-4)^(n-1)`

`T_n = 3(-4)^(n-1)`

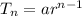
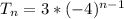
Solving (b): Summation notation.

This implies that the sum of the sequence.

To do this, we write Tn inside the summation sign

i.e.

`\sum\limits^n_1 {T_n}`

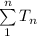
Substitute values for Tn

`\sum\limits^n_1 { 3(-4)^(n-1)`