Question

HELP MATH 100 POINTS HELP US

Answer 1

Let's solve

`\\ \rm\rightarrowtail √(2x-1)-x+2=0`

`\\ \rm\rightarrowtail √(2x-1)=x-2`

`\\ \rm\rightarrowtail 2x-1=(x-2)^2`

`\\ \rm\rightarrowtail 2x-1=x^2-4x+4`

`\\ \rm\rightarrowtail x^2-6x+5=0`

`\\ \rm\rightarrowtail (x-1)(x-5)=0`

• x=1 or 2

Option B is correct

As putting 1 as x

• √1-1+2≠0

Answer 2

Question↷

Which statement best reflects the solution(s) of the equation?

`\small √(2x - 1) - x + 2 = 0`

Answer↷

There is only one solution: x = 5 .

The solution x = 1 is an extraneous solution.

Solution↷

`\small √(2x - 1) - x + 2 = 0`

• Taking (-x+2) to the other side

`\small √(2x - 1) = x - 2`

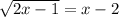
• squaring both of the sides

`\small 2x - 1 = {(x - 2 )}^(2)`

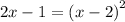
• expanding the sqared binomial as ㅤㅤㅤㅤ(a-b)²= a²- 2ab + b²

`\small 2x - 1 = {x}^(2) - 2 * x * 2 + {2}^(2)`

• simplifying the equation

`\small 2x - 1 = {x}^(2) - 4x + 4`

• asiding the equation

`\small {x}^(2) - 4x - 2x + 4 + 1 = 0\:`

`\small {x}^(2) - 6x + 5 = 0\:`

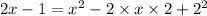
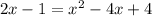
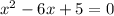
• using splitting middle term method

`\small {x}^(2) - 5x - x+ 5 = 0\:`

`\small x(x- 5) - (x - 5 )= 0\:`

`\small (x- 1)(x - 5 )`

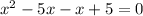
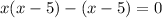
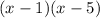
so the root would either be 5 or 1

_____________________________________

putting the value of x as 1,

`\small √(2 * 1 - 1) - 1 + 2 = 0`

`\small 1 - 1 + 2 = 0`

`\small 3 - 1≠ 0`

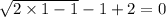
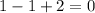
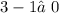
hence , it's not the true solution of the equation

_____________________________________

putting the value of x as 5,

`\small √(2 * 5 - 1) - 5 + 2 = 0`

`\small 3 - 5 + 2 = 0`

`\small 5 - 5 = 0`

`\small 0 = 0`

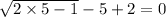
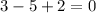
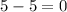
hence ,it's the true solution of the equation