Question

A certain test preparation course is designed to help students improve their scores on the USMLE exam. A mock exam is given at the beginning and end of the course to determine the effectiveness of the course. The following measurements are the net change in 3 students' scores on the exam after completing the course:

15,20,18
Using these data, construct a 90% confidence interval for the average net change in a student's score after completing the course. Assume the population is approximately normal.

Answer 1

The 90% confidence interval for the average net change in a student's score after completing the course is (13.42, 21.92).

Explanation:

Average change in the sample:

`M_(s) = (15+20+18)/(3) = 17.67`

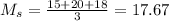
Standard deviation of the sample:

`s = \sqrt{((15-17.67)^2+(20-17.67)^2+(18-17.67)^2)/(2)} = 2.52`

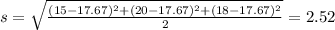
We have the standard deviation for the sample, so the t distribution is used.

The first step to solve this problem is finding how many degrees of freedom, we have. This is the sample size subtracted by 1. So

df = 3 - 1 = 2

90% confidence interval

Now, we have to find a value of T, which is found looking at the t table, with 2 degrees of freedom(y-axis) and a confidence level of
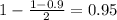`1 - (1 - 0.9)/(2) = 0.95`. So we have T = 2.92

The margin of error is:

`M = T(s)/(√(n)) = 2.92(2.52)/(√(3)) = 4.25`

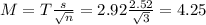
In which s is the standard deviation of the sample and n is the size of the sample.

The lower end of the interval is the sample mean subtracted by M. So it is 17.67 - 4.25 = 13.42

The upper end of the interval is the sample mean added to M. So it is 17.67 + 4.25 = 21.92

The 90% confidence interval for the average net change in a student's score after completing the course is (13.42, 21.92).