Answer:
0.9995 = 99.95% probability that a rivet is defective
Explanation:
For each rivet, there is only two possible outcomes. Either they are defective, or they are not. Rivets are defective independently of one another, each with the same probability, which means that the binomial probability distribution is used to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.
An aircraft seam requires 29 rivets.
This means that

23% of all seams need reworking
This means that
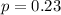
What is the probability that a rivet is defective?
This is:
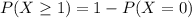
In which

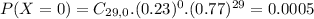
Then
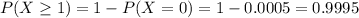
0.9995 = 99.95% probability that a rivet is defective