Question

A 64.0 cm long cord is vibrating in such a manner that it forms a standing wave with two antinodes. (The cord is fixed at both ends.) Which harmonic does this wave represent

Answer 1

the wave represents the second harmonic.

Step-by-step explanation:

Given;

length of the cord, L = 64 cm

The first harmonic of a cord fixed at both ends is given as;

`f_o = (V)/(2L)`

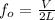
The wavelength of a standing wave with two antinodes is calculated as follows;

L = N---> A -----> N + N ----> A -----> N

Where;

N is node

A is antinode

L = N---> A -----> N + N ----> A -----> N = λ/2 + λ/2

L = λ

The harmonic is calculated as;

`f = (V)/(\lambda) \\\\f = (V)/(L) = 2((V)/(2L) ) = 2(f_o) = 2^(nd) \ harmonic`

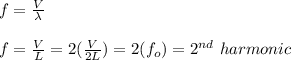
Therefore, the wave represents the second harmonic.

L = λ