Question

What number can you multiply ONE equation by in the following system to obtain a matching

coefficient? (Elimination)
4x+3y=8
2x+5y=12
(Worth 10 points )

Answer 1

Multiply the second equation by 2

Explanation:

Given

`4x + 3y = 8`

`2x + 5y =12`

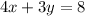
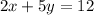
Required

Get matching coefficient for elimination

`4x + 3y = 8`

`2x + 5y =12`

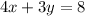
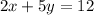
The coefficient of x in both expressions are factors.

So, we can multiply the second equation by 2 to get the coefficient of x in the first.

i.e.

`2 * (2x + 5y = 12)`

`4x + 10y = 24`

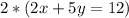
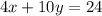
So, the system becomes:

`4x + 3y = 8`

`4x + 10y = 24`

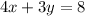
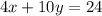
Now, x can be eliminated