Answer:

Explanation:
In a right triangle, the hypotenuse is the longest side and the right angle is the angle opposite the hypotenuse.
Therefore, the hypotenuse is the side with measure 65, and the right angle is the angle formed by the vertex of sides 33 and 56.
The trig ratio for sine is:
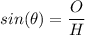
where
 is the angle, O is the side opposite the angle, and H is the hypotenuse.
is the angle, O is the side opposite the angle, and H is the hypotenuse.
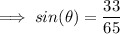
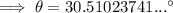
I don't understand the part of the question where it says "leave the answer in simplified radical form". It is very time consuming and laborious to convert a decimal into a radical, and this doesn't really match the level of the initial question of stating the trig ratio.
Please comment below if you need more help/explanation and I will do my best!