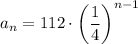Answer:
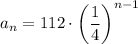
Explanation:
The difference between each term in the sequence is not the same, therefore the sequence is a geometric sequence.
Geometric sequence formula:
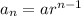
where
 is the start term and
is the start term and
 is the common ratio
is the common ratio
Given

To calculate
 , divide one term by its previous term:
, divide one term by its previous term:
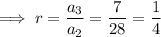
Therefore,