Question

Find the volume of the regular hexagonal pyramid if the lateral edge is 15 feet.

Answer 1

Volume of the given pyramid = 1122.37 cubic feet

Explanation:

Volume of the regular hexagonal pyramid =
`(1)/(3)(\text{Area of the base})(\text{Base})`

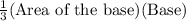
Measure of internal angle of a polygon =
`((n-2)* 180)/(n)`

Here, n = number of sides of the polygon

For a hexagon, n = 6

Measure of interior ∠C =
`((6-2)* 180)/(6)`

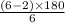
= 120°

Measure of ∠BCD =
`(120)/(2)`

= 60°

By applying tangent rule in ΔCED,

tan(∠ECD) =
`\frac{\text{Opposite side}}{\text{Adjacent side}}`

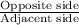
tan(60°) =
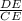
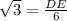
`(DE)/(CE)`

`√(3)=(DE)/(6)`

DE =
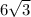`6√(3)` feet

And cos(60°) =
`(EC)/(CD)`

`(1)/(2)=(6)/(CD)`

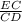
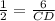
CD = 12 feet

Area of ΔBCD =
`(1)/(2)(\text{Base})(\text{Height})`

=
`(1)/(2)(6√(3))(12)`

=
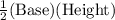
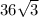`36√(3)` feet

Area of hexagonal Base of the pyramid =
`6(36√(3))`

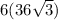
= 216√3 square feet

Since, lateral height of the pyramid (AC) = 15 feet

By applying Pythagoras theorem in ΔADC,

AC² = AD² + CD²

(15)² = AD² + (12)²

AD =
`√(225-144)`

AD = 9 feet

Volume of the given pyramid =
`(1)/(3)(216√(3))(9)`

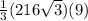
= 648√3 cubic feet

= 1122.37 cubic feet