Question

Please help me find the answer to this question

Answer 1

-------------------------------------------------------------------------------------------------------------

Answer: The top right table

-------------------------------------------------------------------------------------------------------------

Given:
`\textsf{y = 30 - 3x}`

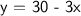
Find:
`\textsf{Match the table of values with the equation}`

Solution: First we must understand what form our equation is in and what each of the numbers represent. This form is known as the slope intercept form which is formatted as y = mx + b. Our equation is slightly re-ordered but we can change that by converting it to y = -3x + 30 where m is equal to -3 and b is equal to 30. Let us know talk about what m and b are. M is the slope of the equation or also known as the rise/run or the change in y over the change in x. B is the y-intercept which is basically the value of y when x is equal to 0. In this case, our b is equal to 30.

Looking at the tables we can see what the value of y when x is equal to 0 for each table. The only table that matches up stating that y is equal to 30 when x is 0 is the top right table.

You do not have to go any further but if you want to confirm that the slope is as expected we can do the following:
`(y_2\ -\ y_1)/(x_2\ -\ x_1)`.

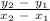Plug in the values and simplify

• 
  `(48\ -\ 30)/(-6\ -\ 0)`

• 
  `(18)/(-6)`

• 
  `-3`

Therefore, we can confirm that the slope matches up with the given equation as well. So, the final answer would be that the top right table would correspond to the equation y = 30 - 3x.