Answer:
10521₈
Explanation:
First convert the number from base-7 (septenary) to base-10 (decimal):
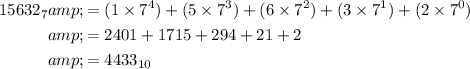
Now convert it from base-10 (decimal) to base-8 (octal).
Divide 4433 by 8 repeatedly until you arrive at a quotient less than 8.
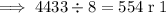

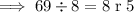

Arrange the final quotient and the remainders of all the divisions in the bottom-to-top direction: