Answer:
No (not a proportional relationship)
Explanation:
The cost of fountain drinks at Hot Dog Hut:
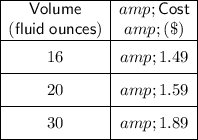
A proportional relationship is one in which two quantities vary directly with each other.
Calculate the rate of change of the ordered pairs.
Rate of change between (30, 1.89) and (20, 1.59):
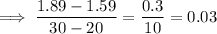
Rate of change between (20, 1.59) and (16, 1.49):
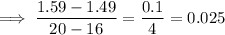
As the rates of change are different (not constant), the relationship is not proportional.
Note: The graph of a proportional linear relationship is a line that passes through the origin (0, 0).