Question:-
A cylinder has a radius of 4 inches and a height of 5 inches. If the radius of the cylinder is tripled and the height remains the same to create a new cylinder, what will be the difference in the surface areas of the two cylinders ? Answer in terms of Pi.
Answer:-
Given:-
A cylinder has a radius of 4 inches and a height of 5 inches.
To Find:-
If the radius of the cylinder is tripled and the height remains the same to create a new cylinder, what will be the difference in the surface areas of the two cylinders ?
Solution:-
Let in case of 1st cylinder,
 Radius
Radius
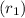 is 4 inches
is 4 inches
 Height is 5 inches
Height is 5 inches
And in case of 2nd cylinder,
 Radius
Radius
 is 3 × 4 = 12 inches [as in the question it is given that the radius of the cylinder is tripled]
is 3 × 4 = 12 inches [as in the question it is given that the radius of the cylinder is tripled]
 Height is 5 inches
Height is 5 inches
Now,
Surface area
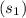 of 1st cylinder
of 1st cylinder
is 2πr(r + h) = 2π × 4(4 + 5)
= 8π(9)
= 72π sq inches
Surface area
 of 2nd cylinder
of 2nd cylinder
is 2πr(r + h) = 2π × 12(12 + 5)
= 24π(17)
= 408π sq inches
 The difference in the surface areas of the two cylinders is =
The difference in the surface areas of the two cylinders is =
 = (408π - 72π) = 336π sq inches
= (408π - 72π) = 336π sq inches
The difference in the surface areas of the two cylinders is 336π sq inches. [Answer]