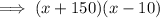Question

Where do u get the 150 out of the answer (x+150 (x-10) in the equation x^2+140x-1500?

Answer 1

See explanation.

Explanation:

Given quadratic:

`x^2+140x-1500`

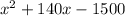
To factor a quadratic in the form
`ax^2+bx+c`, first find two numbers that multiply to
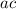`ac` and sum to
`b`.

As a = 1, b = 140 and c = -1500, then:

`\implies a \cdot c=1 \cdot -1500=-1500`

`\implies b=140`

Therefore, the two numbers are 150 and -10 as:

`\implies 150 \cdot -10 = -1500`

`\implies 150+(-10)=140`

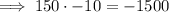
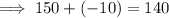
Rewrite
`b` as the sum of these two numbers:

`\implies x^2+(150-10)x-1500`

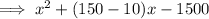
Distribute:

`\implies x^2+150x-10x-1500`

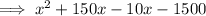
Factor the first two terms and the last two terms separately:

`\implies x(x+150)-10(x+150)`

Factor out the common term (x + 150):

`\implies (x+150)(x-10)`