Question

25 points!!!!!!!!!!!!!!!!!!

The length of the hypotenuse of 30 - 60 - 90 a triangle is 6. Find the perimeter. Round answer to the nearest hundredth.

Answer 1

14.2

Explanation:

Hi there!

We are given a 30-60-90 triangle

The triangle is a right triangle, since one of the angles measures 90 degrees

We are also given that the length of the hypotenuse (the side OPPOSITE from the right angle) is 6

We want to find the perimeter of the triangle

There are special formulas to find the other sides of the triangle, if we know that:

a. the triangle is a right triangle

b. we know the length of the hypotenuse

These two formulas are:

• If the length of the hypotenuse in a triangle is a, then the length of the side OPPOSITE from the 30 degree angle is
  `(a)/(2)`

• The length of the side OPPOSITE from the 60 degree angle is
  `(a√(3) )/(2)`

In this problem, we are given that a=6, so that means that in order to find the length of the other sides:

The length of the side opposite from the 30° angle:
`(a)/(2) = (6)/(2) = 3`

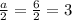
The length of the side opposite from the 60° angle:
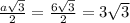`(a√(3) )/(2) = (6√(3) )/(2) = 3√(3)`, or about 5.2

Now, to find the perimeter, we add the lengths of these sides together.

6 + 3 + 5.2 = 14.2

Hope this helps!