Question

Find the distance between the points (2, – 5) and ( – 7, – 7)

Answer 1

=
`√(85)`

Explanation:

let, (x1,y1) = (2,-5) & (x2,y2) = (-7,-7)

now, for distance between two points,

D =
`\sqrt[]{(x_(1)-x_(2) )^(2) +(y_(1)-y_(2) )^(2) }`

=
`\sqrt[]{(2-(-7))^(2)+(-5-(-7))^2 }`

`=√((2+7)^2+(-5+7)^2)`

`=√(9^2+2^2)`

`=√(81+4)`

`=√(85)`

Answer 2

`\huge{ \boxed{ √(85) \: \text{or} \: 9.219 \: \text{units}}}`

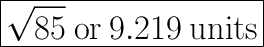
Explanation:

The distance between two points say
`A(x_1,y_1)` and B(x_2,y_2) can be found by using the formula;

`\bold{d = \sqrt{{x_2-x_1}^(2)+{y_2-y_1}^(2)}}`

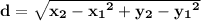
From the question the points are (2, – 5) and ( – 7, – 7)

`x_1=2 \\ y_1=-5 \\ x_2=-7 \\ y_2=-7`

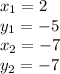
Substituting the values into the formula that is;

`d = \sqrt{ {( - 7 - 2)}^(2) + {( - 5 - - 7)}^(2) } \\ d = \sqrt{ {( - 9)}^(2) + {(2)}^(2) } \\ d = √(81 + 4) \\ d = √(85) = 9.219`

We have the final answer as

√85 or 9.219 units