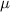Question

Population 1,2,4,5,8 · Draw all possible sample of size 2 W.O.R · Sampling distribution of Proportion of even No. · Verify the results

Answer 1

Question:

A population consists 1, 2, 4, 5, 8. Draw all possible samples of size 2 without replacement from this population.

Verify that the sample mean is an unbiased estimate of the population mean.

Answer:

`Samples: \{(1,2),(1,4),(1,5),(1,8),(2,4),(2,5),(2,8),(4,5),(4,8),(5,8)\}`

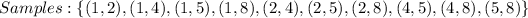
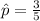`\hat p = (3)/(5)` --- proportion of evens

The sample mean is an unbiased estimate of the population mean.

Explanation:

Given

`Numbers: 1, 2, 4, 5, 8`

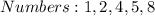
Solving (a): All possible samples of 2 (W.O.R)

W.O.R means without replacement

So, we have:

`Samples: \{(1,2),(1,4),(1,5),(1,8),(2,4),(2,5),(2,8),(4,5),(4,8),(5,8)\}`

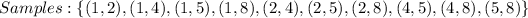
Solving (b): The sampling distribution of the proportion of even numbers

This is calculated as:

`\hat p = (n(Even))/(Total)`

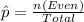
The even samples are:

`Even = \{2,4,8\}`

`n(Even) = 3`

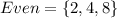
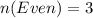
So, we have:

`\hat p = (3)/(5)`

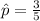
Solving (c): To verify

`Samples: \{(1,2),(1,4),(1,5),(1,8),(2,4),(2,5),(2,8),(4,5),(4,8),(5,8)\}`

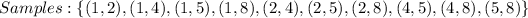
Calculate the mean of each samples

`Sample\ means = \{1.5,2.5,3,4.5,3,3.5,5,4.5,6,6.5\}`

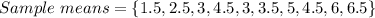
Calculate the mean of the sample means

`\bar x = (1.5 + 2.5 +3 + 4.5 + 4 + 3.5 + 5 + 4.5 + 6 + 6.5)/(10)`

`\bar x = (40)/(10)`

`\bar x = 4`

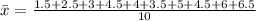
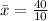
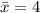
Calculate the population mean:

`Numbers: 1, 2, 4, 5, 8`

`\mu = (1 +2+4+5+8)/(5)`

`\mu = (20)/(5)`

`\mu = 4`

`\bar x = \mu = 4`

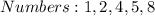
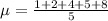
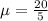
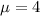
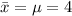
This implies that
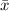`\bar x` is an unbiased estimate of the
`\mu`