Question

The diagram shows a rectangle and a diagonal of the rectangle. Length=8.5cm. Width=5.6cm. Work out the length of the diagonal of the rectangle. Give your answer correct to 1 decimal place

Answer 1

10.2cm

Explanation:

Diagonal= √8.5^2 + 5.6^2

=10.2cm

Answer 2

The length of the diagonal of the rectangle is approximately 9.9 cm.

To find the length of the diagonal of a rectangle, we can use the Pythagorean Theorem, which states that in a right-angled triangle, the square of the length of the hypotenuse (in this case, the diagonal) is equal to the sum of the squares of the other two sides.

Let the length of the rectangle be 8.5 cm, the width be 5.6 cm, and the diagonal be d.

Applying the Pythagorean Theorem:

d^2 =8.5^2 +5.6^2

d^2 =72.25+31.36

d^2 =103.61

Taking the square root of both sides to solve for d:

d≈
`√(103.61)`

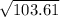
​d≈10.18cm

Rounding to one decimal place, the length of the diagonal of the rectangle is approximately 10.2 cm.