Question

The car that Diana bought is 8 years old. She paid $6,700. This make and model depreciates exponentially at a rate of 14.15% per year . What was the original price of the car when it was new?

Answer 1

Answer:261,400

Explanation:

Answer 2

The original price of the car when it was new was approximately $2,160.84.

The formula for exponential depreciation is given by:

`\[ P(t) = P_0 \cdot e^(rt) \]`

Where:

-
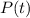`\( P(t) \)` is the current value of the asset at time
`\( t \)`,

-
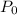`\( P_0 \)` is the original value of the asset,

-
`\( r \)` is the annual depreciation rate (expressed as a decimal),

-
`\( t \)` is the time in years,

-
`\( e \)` is the mathematical constant approximately equal to 2.71828.

In this case, the car is 8 years old, the current value is $6,700, and the depreciation rate is 14.15% per year.

Let's substitute these values into the formula:

`\[ 6700 = P_0 \cdot e^((0.1415 \cdot 8)) \]`

Now, solve for
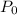`\( P_0 \)`, the original price:

`\[ 6700 = P_0 \cdot e^(1.132) \]`

To solve for
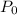`\( P_0 \)`, divide both sides by
`\( e^(1.132) \)`:

`\[ P_0 = (6700)/(e^(1.132)) \]`

Using a calculator:

`\[ P_0 \approx (6700)/(e^(1.132)) \]`

`\[ P_0 \approx (6700)/(3.1009) \]`

`\[ P_0 \approx 2160.84 \]`

Therefore, the original price of the car when it was new was approximately $2,160.84.