Answer:
Hypotenuse = 159 ft (nearest integer)
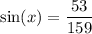
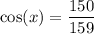
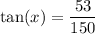
Explanation:

From inspection of the given right triangle:
Substitute these values into Pythagoras Theorem and solve for c (hypotenuse):
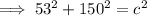




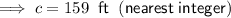
Therefore, the hypotenuse of the given triangle is 159 ft (nearest integer).
-----------------------------------------------------------------------------------------------
Trigonometric ratios

where:
- θ is the angle.
- O is the side opposite the angle.
- A is the side adjacent the angle.
- H is the hypotenuse (the side opposite the right angle).
Given:
- θ = x
- A = 150
- O = 53
- H = 159
Substitute the values into the ratios:
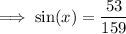


SOHCAHTOA is a mnemonic for the definitions of the trigonometric ratios applicable to right triangles:
- Sine of an angle is equal to Opposite over Hypotenuse.
- Cosine of an angle is equal to Adjacent over Hypotenuse.
- Tangent of an angle is equal to Opposite over Adjacent.
(See attachment).