Problem 1:
![sin(75^o)=sin(45^o+30^o)\\sin(A+B)=sinAcosB+cosAsinB\\sin(45^o+30^o)=sin45^ocos30^o+cos45^osin30^o\\(1)/(√(2) ) *(√(3) )/(2) +(1)/(√(2) ) ]*(1)/(2) \\(√(3) )/(2√(2) ) +(1)/(2√(2) ) ---- > (√(3)+1)/(2√(2) ) (ANS)](https://img.qammunity.org/2023/formulas/mathematics/high-school/83b6xrtqdd8lnunrdz7op0t3xu4hcbllr5.png)
Problem 2:
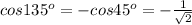
Step-by-step explanation:
Cos 135° is an angle in the second quadrant.
In the second quadrant, cos is negative.
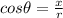
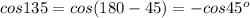
An angle of 45° is found in a right-angled triangle of sides
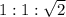
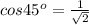
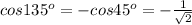
Note that
 is an irrational number and cannot be given as an exact decimal.
is an irrational number and cannot be given as an exact decimal.
Little messy math, but thanks for asking the question.
- Eddie