Answer:
20 hours worked at the local library.
25 hours worked as a personal trainer.
Explanation:
Definition of the variables:
- Let x = number of hours worked at the local library.
- Let y = number of hours worked as a personal trainer.
Given information:
- Working at the local library pays $15 per hour.
- Working as a personal trainer pays $20 per hour.
- Last week you made $800 and worked 45 total hours.
Create a system of equations using the defined variables and the given information:
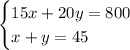
Rearrange the second equation to make y the subject:
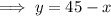
Substitute this into the first equation and solve for x:
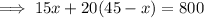
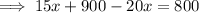
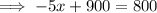

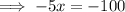
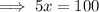
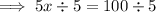

Substitute the found value of x into the second equation and solve for y:
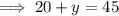
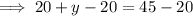
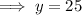
Therefore:
- 20 hours worked at the local library.
- 25 hours worked as a personal trainer.