Question

Consider this right triangle.
Enter the ratio equivalent to sin (B)

Answer 1

`\boxed {\boxed {\sf sin(B)= \frac {21}{29}}}`

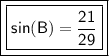
Explanation:

Sine is the ratio of the opposite side to the hypotenuse.

`sin \theta= \frac {opposite}{hypotenuse}`

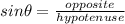
We want to find the sine of angle B. The side AC, which measures 21, is opposite angle B.

The side AB, which measures 29, is the hypotenuse because it is the longest side and opposite the right angle.

`opposite= 21\\hypotenuse=29`

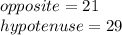
Substitute the values into the formula.

`sinB= \frac {21}{29}`

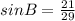
This ratio cannot be reduced further, so it is the final answer.

The sine of B is 21/29