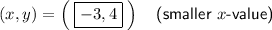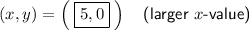Question

asked May 1, 2023 190k views

2 Answers

Gralex · Answer 1 · 2023-05-03T10:09:29+0000

Answer:

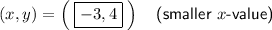
$(x,y)=\left(\; \boxed{-3,4} \; \right)\quad \textsf{(smaller $x$-value)}$

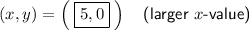
$(x,y)=\left(\; \boxed{5,0} \; \right)\quad \textsf{(larger $x$-value)}$

Explanation:

Given system of equations:

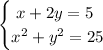
$\begin{cases}\;x+2y=5\\x^2+y^2=25\end{cases}$

To solve by the method of substitution, rearrange the first equation to make x the subject:

$\implies x=5-2y$

Substitute the found expression for x into the second equation and rearrange so that the equation equals zero:

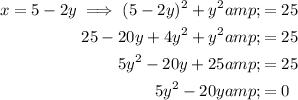
$\begin{aligned}x=5-2y \implies (5-2y)^2+y^2&=25\\25-20y+4y^2+y^2&=25\\5y^2-20y+25&=25\\5y^2-20y&=0\end{aligned}$

Factor the equation:

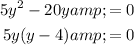
$\begin{aligned}5y^2-20y&=0\\5y(y-4)&=0\end{aligned}$

Apply the zero-product property and solve for y:

$5y=0 \implies y=0$

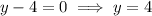
$y-4=0 \implies y=4$

Substitute the found values of y into the first equation and solve for x:

$\begin{aligned}y=0 \implies x+2(0)&=5\\x&=5\end{aligned}$

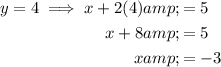
$\begin{aligned}y=4 \implies x+2(4)&=5\\x+8&=5\\x&=-3\end{aligned}$

Therefore, the solutions are:

$(x,y)=\left(\; \boxed{-3,4} \; \right)\quad \textsf{(smaller $x$-value)}$

$(x,y)=\left(\; \boxed{5,0} \; \right)\quad \textsf{(larger $x$-value)}$

Pepero · Answer 2 · 2023-05-08T01:05:23+0000

Answer:

=====================

Please log in or register to add a comment.