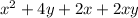
We can factorise this expression by grouping. First let us arrange it in this way
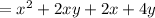
Let us bracket them like this:
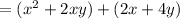
In the first bracket portion, take x as common and in the second expression, take 2 as common.
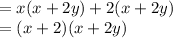
Answer:
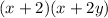
Hope you could understand.
If you have any query, feel free to ask.