Answer:
There is not sufficient evidence at the 0.01 level that the bags are underfilled or overfilled
Explanation:
A manufacturer of chocolate chips would like to know whether its bag filling machine works correctly at the 403 gram setting.
This means that the null hypothesis is:
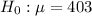
Is there sufficient evidence at the 0.01 level that the bags are underfilled or overfilled:
This means that at the alternate hypothesis, we are testing if the mean is different than 403, that is:
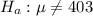
The test statistic is:
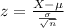
In which X is the sample mean,
 is the value tested at the null hypothesis,
is the value tested at the null hypothesis,
 is the standard deviation and n is the size of the sample.
is the standard deviation and n is the size of the sample.
Value of 403 tested at the null hypothesis:
This means that
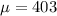
A 10 bag sample had a mean of 411 grams with a variance of 121.
This means that
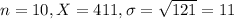
Value of the test statistic:
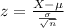
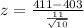
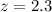
Pvalue:
Testing if the mean is different of a value, and z positive, which means that the pvalue is 2 multiplied by 1 subtracted by the pvalue of z = 2.3
Looking at the z-table, z = 2.3 has a pvalue of 0.9893
1 - 0.9893 = 0.0107
2*0.0107 = 0.0214
0.0214 > 0.01, which means that there is not sufficient evidence at the 0.01 level that the bags are underfilled or overfilled