Question

The length of a rectangle is increasing at a rate of 9 cm/s and its width is increasing at a rate of 4 cm/s. When the length is 15 cm and the width is 12 cm, how fast is the area of the rectangle increasing

Answer 1

The area of the rectangle is increasing at a rate of 168 square centimeters per second.

Explanation:

Geometrically speaking, the area of a rectangle (
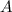`A`), in square centimeters, is described by following expression:

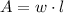`A = w\cdot l` (1)

Where:

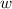`w` - Width, in centimeters.

`h` - Height, in centimeters.

By Differential Calculus, we find an expression for the rate of change of the area of the rectangle (
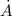`\dot A`), in square centimeters per second:

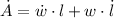`\dot A = \dot w\cdot l + w\cdot \dot l` (2)

Where:

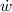`\dot w` - Rate of change of the width of the rectangle, in centimeters per second.

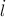`\dot l` - Rate of change of the length of the rectangle, in centimeters per second.

If we know that
`w = 12\,cm`,
`l = 15\,cm`,
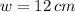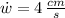`\dot w = 4\,(cm)/(s)` and
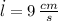`\dot l = 9\,(cm)/(s)`, then the rate of change of the area of the rectangle is:

`\dot A = \dot w\cdot l + w\cdot \dot l`

`\dot A = 168\,(cm^(2))/(s)`

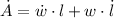
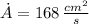
The area of the rectangle is increasing at a rate of 168 square centimeters per second.