Given: Many electronics follow a failure rate described by an exponential probability density function (PDF). Solar panels are advertised to last 20 years or longer, but panels made in China are failing at a higher rate. The time-to-failure of this device is usually exponentially distributed, with a mean of 12 years.
Required: To determine the probability of failure in the first 8 years.
Explanation: Acording to the question,
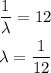
As we know,
The cumulative distributive function will be:
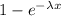
Hence, In the first 8 years the probability of failure is

Final Answer: The probability of failure in the first 8 years is 0.4865.