Solution:
Given:
In order for matrix multiplication to be defined, the number of columns in the first matrix must be equal to the number of rows in the second matrix.
Also, in order for matrix addition to be possible, the two matrices must have the same dimensions.
Hence,

Thus the matrices operations that are not possible are;
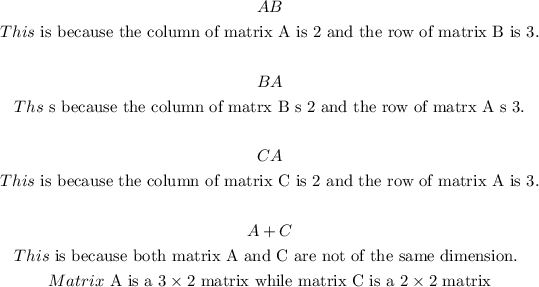
All other operations are possible because, for the multiplied matrices, the number of columns of the first matrix is equal to the number of rows of the second matrix.
Also, the added matrices are possible because the two added matrices are of the same order.
Therefore, the matrix operations that are not possible are;