Answer:
Explanations:
Given the following parameters;
Mean = 78
Standard deviation = 4
If a randomly selected student with a score between 71 and 85, then we need the probability P(71
Determine the z-score for each score
For a score of 71
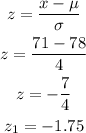
For a score of 85
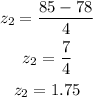
Determine the probability for the z-score values P(-1.75 < z < 1.75) using the standard table.
[tex]\begin{gathered} P(-1.75Hence the
percentage of scores for a randomly selected student with a
score between 71 and 85 is 91.98%