Given:
The potential difference V=3000 V
The radius of curvature of the curved path of the electron, r=26.0 cm=0.26 m
The velocity of the electron, v=32.5 Mm/s=32.5×10⁶ m/s
To find:
The magnitude of the magnetic field.
Step-by-step explanation:
The magnetic force acting on the electron provides it the neccessary centripetal force to trace a curved path.
Thus the centripetal force acting on the electron is equal to the magnetic force acting on it.
Therefore,
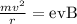
Where m is the mass of the electron, e is the charge of the electron, and B is the magnitude of the magnetic field.
On rearranging the above equation,
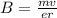
On substituting the known values,
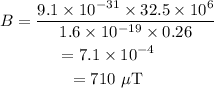
Final answer:
The magnitude of the magnetic field is 710 μT.