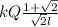Given:
Three-point charges are placed at the three corners of the square of side l.
The charges are respectively,
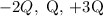
To find:
The potential at the fourth corner
Step-by-step explanation:
The distance between the opposite corners is,
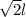
The potential at any point at a distance r, from a charge q is,
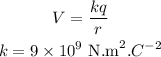
For the charges in the three corners, the potential at the fourth corner is,
![\begin{gathered} V=k[(-2Q)/(l)+(Q)/(√(2)l)+(3Q)/(l)] \\ =k(-2√(2)Q+Q+3√(2)Q)/(√(2)l) \\ =kQ(1+√(2))/(√(2)l) \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/opqvbbliwtkyt4hscjbudutstib6lb5mcz.png)
Hence, the potential at the fourth corner is,