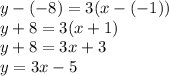Answer:
y = 3x - 5
Explanation:
1) First, place the given equation in slope-intercept form, represented by the formula
 , to find the slope. Whatever
, to find the slope. Whatever
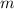 or the coefficient of x-term is will be the slope. Isolate y in the equation:
or the coefficient of x-term is will be the slope. Isolate y in the equation:

So, the slope of that line is
 . Lines that are perpendicular have slopes that are opposite reciprocals, thus the slope of the new line will be 3.
. Lines that are perpendicular have slopes that are opposite reciprocals, thus the slope of the new line will be 3.
2) Now, use the point-slope formula
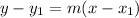 to find the equation of the new line. Substitute real values for the
to find the equation of the new line. Substitute real values for the
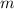 ,
,
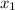 and
and
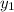 in the equation.
in the equation.
Since
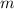 represents the slope, substitute
represents the slope, substitute
 in its place. Since
in its place. Since
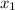 and
and
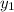 represents the x and y values of one point the line passes through, substitute the x and y values of (-1,-8) into the formula as well. Then, isolate the y in the resulting equation to put the equation in slope-intercept form and find an answer:
represents the x and y values of one point the line passes through, substitute the x and y values of (-1,-8) into the formula as well. Then, isolate the y in the resulting equation to put the equation in slope-intercept form and find an answer: