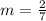Question

Find the slope of the line that passes through (-31, 26) and (4, 36).

Answer 1

The slope of a line can be calculated with the following formula:

`m=(y_2-y_1)/(x_2-x_1)`

You know that the line passes through the following points:

`\mleft(-31,26\mright);(4,36)`

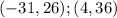
For this case, you can set up that:

`\begin{gathered} y_2=36 \\ y_1=26 \\ x_2=4 \\ x_1=-31 \end{gathered}`

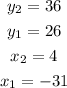
Then, knowing the coordinates shown above, you can substitute them into the formula in order to find the slope of the line. This is:

`\begin{gathered} m=(36-26)/(4-(-31)) \\ \\ m=(10)/(35) \\ \\ m=(2)/(7) \end{gathered}`

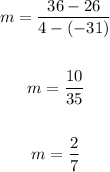
The answer is:

`m=(2)/(7)`